Where art and science meet
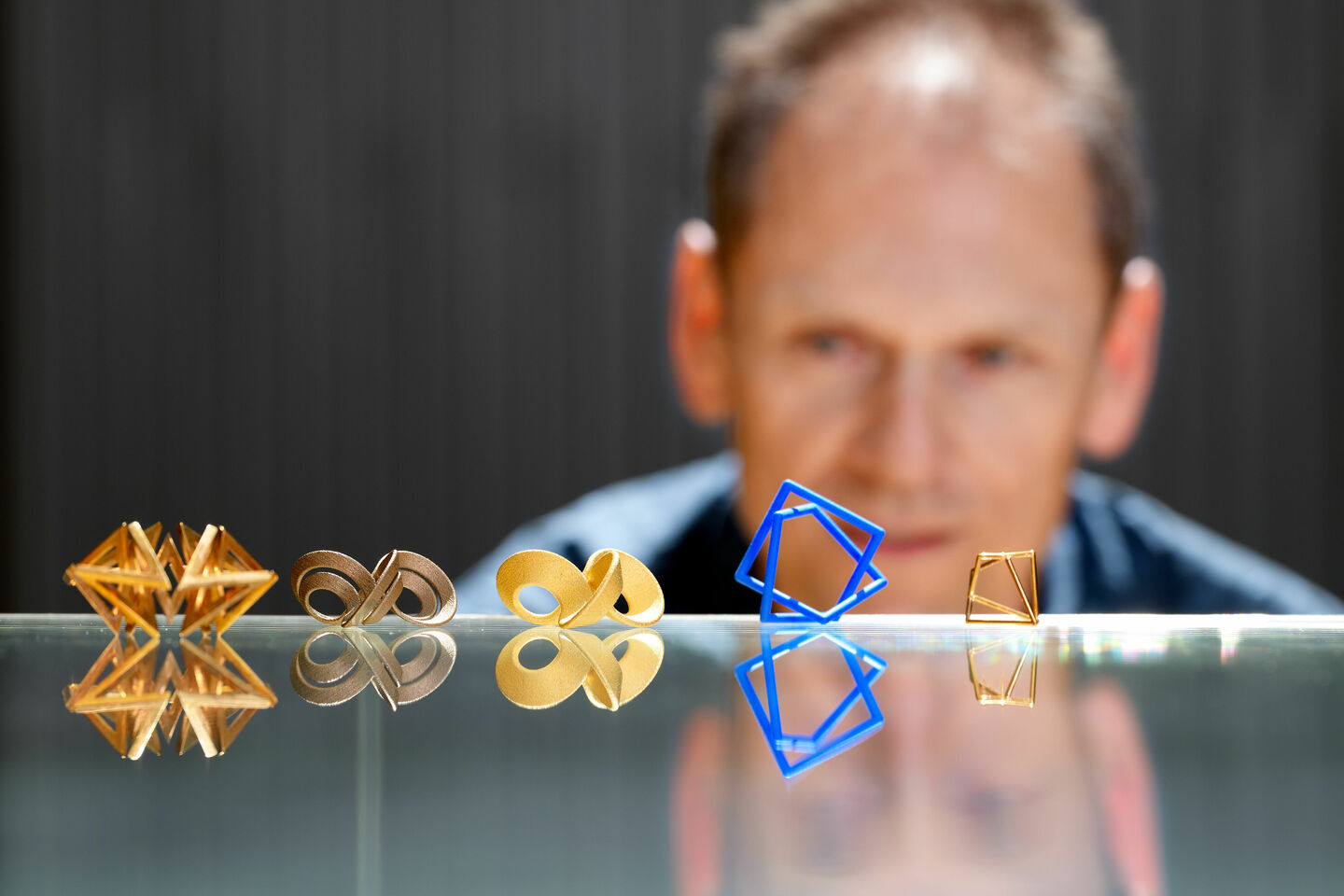
The mathematical art in the elevator space in MetaForum can fool you a little. Bamboozle, by Koos and Tom Verhoeff, was designed to fool and obscure the crowd. “It was quite a challenge for us to identify the mathematical structures in this form. It’s a strange thin structure, quite rare.” Tom Verhoeff, researcher at the department of Mathematics and Computer Science, likes to spend his free time on creating beauty by combining the fields of mathematics and art: mathematical art.
“I was inspired by my father Koos Verhoeff to explore mathematical art. My father, who also was a mathematician, had been working on mathematical art pieces for quite some time. Because I grew up in a mathematical environment, I developed a sense for beauty in mathematics from an early age. You can hardly be a mathematician if you lack that sense for beauty and elegance within mathematics, in my opinion. You can solve something or you can solve something beautifully. A real mathematician aims for the latter.”
You can solve something or you can solve something beautifully. A real mathematician aims for the latter
“What fascinates me about mathematical art is its elegance. That you can be working with beautiful things. Mathematical art is strongly extrovert, it communicates something but in a very different way than a classic presentation does. A piece of art needs to be able to do it on its own, tell its own story. Here I see a similarity with education, to some extent. You can tell students a lot, but the real click, the insight, can be realized with something very simple. Through very concrete examples. Making something that is sufficient to convey a certain idea. Art also faces that challenge."
“When I was in college, I was totally focused on my education. I was not interested in art. Ten years ago I decided to record the not immediately visible mathematics in my father’s art, as he didn’t write much about the works of art he had made. Then I started to write scientific articles to capture the ideas behind the most important works and shared these with the world. That was mainly analyzing, but sometimes new things came out of the process. And I made suggestions to my father. The Bamboozle was something we created together, a piece of synergy.”
“There is a lot of hidden mathematics in art that you do not see, but that is important. Mathematical art makes mathematics visible, makes the abstraction concrete. You really have to think in a problem-solving way. That also means that it is actually a very creative profession. Such beautiful things are done in mathematics, that we want non-mathematicians to enjoy them as well.”
Bach
“Art can originate from mathematics without ever being intended as art. On the other hand, you have artists who use mathematical things with the intention of making art. Mathematical techniques are also used in the analysis when studying 'ordinary art'. For example with the music of Bach. He was not a mathematician and music is not mathematical. But there are structures that are mathematical. Mathematicians recognize such patterns, like symmetry. Artists who use these kinds of things play with mathematical elements, visible or less visible and consciously or less consciously. The combination of these elements is mathematical art to me.”
"Not everything in a piece of art needs to be mathematically relevant, but it should be artistic. It keeps your attention, it intrigues you. It is there and it is beautiful. That is for me as a mathematician, art. In addition to a mathematical aspect, there must also have been an artistic struggle. That makes it art, because it does not happen by itself. The artist must have thought about what message it conveys to the material world. It has to be beautiful, it has to raise questions, but it also needs to be of a simple nature.”
In addition to a mathematical aspect, there must also have been an artistic struggle. That makes it art, because it does not happen by itself
Celebrating Mathematics
Want to know more about mathematical art and how mathematics is hidden in art? Verhoeff will lecture during the Celebrating Mathmatics conference. His presentation is called 'Mathematical art as problem solving'. This will take place on the 27th of August at TU/e.






Discussion